When it comes to geometry, parallelograms and circles are common shapes that are studied extensively. One interesting and useful concept in geometry is the fact that when a circle is inscribed within a parallelogram, the parallelogram formed by connecting the midpoints of the sides is a rhombus. This concept is crucial for understanding the properties and relationships between different geometric shapes. In this article, we will delve into the details of how a parallelogram circumscribing a circle is actually a rhombus, exploring the definitions, properties, and proofs that support this intriguing relationship.
Understanding the Basics: Parallelograms and Circles
Before we delve into the relationship between a parallelogram, a circle, and a rhombus, let’s quickly review the basic properties of these shapes:
Parallelogram:
– A parallelogram is a quadrilateral with opposite sides that are parallel and equal in length.
– The opposite angles of a parallelogram are also equal.
Circle:
– A circle is a set of points in a plane that are equidistant from a given point, known as the center of the circle.
– The distance from the center of the circle to any point on the circle is the radius of the circle.
The Relationship: Parallelogram Circumscribing a Circle
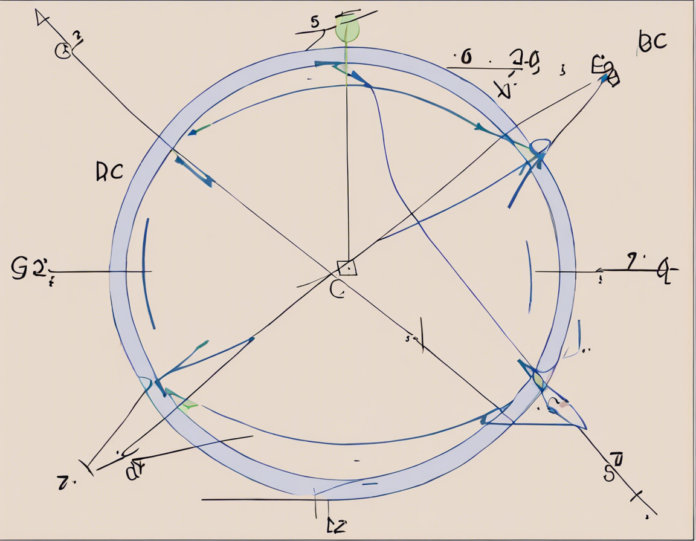
Now, let’s explore the relationship between a parallelogram and a circle. When a circle is inscribed within a parallelogram, it touches the sides of the parallelogram at four points. By connecting the midpoints of these sides, we can form a new quadrilateral, as shown in the diagram below.
Properties of the Rhombus
Having established the connection between a parallelogram circumscribing a circle and a rhombus, let’s take a closer look at the properties of a rhombus to understand why this relationship holds true:
1. All Sides are Equal:
In a rhombus, all four sides are equal in length. This property is a direct result of the fact that the circle inscribed within the parallelogram touches the sides at their midpoints, creating segments of equal length.
2. Diagonals are Perpendicular:
The diagonals of a rhombus are perpendicular to each other. This property can also be observed in the quadrilateral formed by connecting the midpoints of the sides of the parallelogram.
3. Diagonals are Bisectors:
The diagonals of a rhombus bisect each other at right angles. This property is a consequence of the circle inscribed within the parallelogram touching the sides at their midpoints.
4. Opposite Angles are Equal:
Similar to a parallelogram, the opposite angles of a rhombus are equal. This property is carried over from the original parallelogram configuration.
5. Symmetry:
A rhombus exhibits symmetry along its diagonals, with each diagonal dividing the rhombus into two congruent triangles.
Proof of the Relationship
To visually understand why a parallelogram circumscribing a circle forms a rhombus, we can consider the following proof:
- Let ABCD be a parallelogram with a circle inscribed within it, touching the sides at points E, F, G, and H.
- Connect the midpoints of the sides of the parallelogram to form the quadrilateral EFGH.
- Since ABCD is a parallelogram, AD is parallel to BC, and AB is parallel to CD.
- By connecting the midpoints of the sides, we create another parallelogram. Therefore, EF is parallel to GH, and EH is parallel to FG.
- In the quadrilateral EFGH, EF is equal to GH, FH is equal to HG, and EH is equal to FG.
- By definition, a quadrilateral with sides of equal length is a rhombus. Therefore, EFGH is a rhombus.
FAQs: Frequently Asked Questions
Here are some commonly asked questions about the concept of a parallelogram circumscribing a circle and forming a rhombus:
Q1: What is the significance of a rhombus in geometry?
A rhombus is a special type of quadrilateral with unique properties, such as having all sides equal in length and diagonals that bisect each other at right angles. Its symmetrical nature makes it a fundamental shape in geometric studies.
Q2: How can we prove that a parallelogram circumscribing a circle is a rhombus?
By connecting the midpoints of the sides of the parallelogram where a circle is inscribed, we form a quadrilateral with sides of equal length. This configuration fulfills the definition of a rhombus, thus proving the relationship.
Q3: Does every parallelogram that circumscribes a circle form a rhombus?
Yes, any parallelogram that has a circle inscribed within it and connects the midpoints of its sides forms a rhombus due to the equal lengths of the segments created by the circle touching the sides.
Q4: What are the practical applications of understanding the relationship between a parallelogram, a circle, and a rhombus?
Understanding these geometric relationships can be useful in various fields, such as architecture, engineering, and design, where precise measurements and symmetrical shapes play a significant role.
Q5: How does the concept of a rhombus within a parallelogram relate to other geometric principles?
The relationship between a parallelogram circumscribing a circle and forming a rhombus demonstrates the interconnectedness of various geometric concepts, highlighting the symmetry and properties of different shapes.
In conclusion, the relationship between a parallelogram circumscribing a circle and forming a rhombus is a fascinating concept in geometry that showcases the interplay between different shapes and their properties. By understanding this relationship and the properties of a rhombus, we can deepen our knowledge of geometric principles and appreciate the beauty of mathematical relationships in the world around us.